A Second Sample Question for Test #1

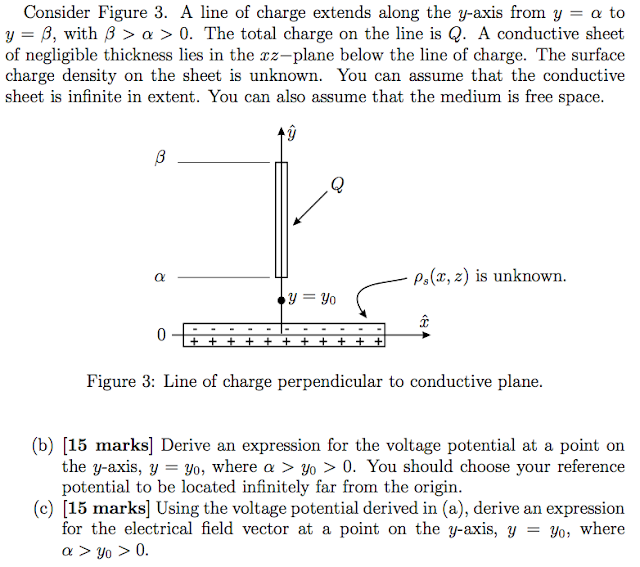
Here's a second question to try. This one you should be able to do right now. Expect something similar on the test.

Solution:

I solved this problem using Mathematica. I solved for the voltage at any point above the surface of the conductor, and below the end of the rod, using this expression:

Note that this is more difficult than answering the question as asked, which limited the position to being directly under the rod.

Here is a contour plot of the voltage for an area under the rod, where I've assumed that the rod extends from 10m to 12m above the surface. These contour lines are equipotential lines.

To get the formula for the electrical field, I found the gradient components in the x and y axes:

The electrical field is the negative of the gradient, which is plotted below:

One more thing we can find is the surface charge density for the conductor surface. This can be found using Gauss's Law (see your notes), from which we get E=rho_s/epsilon_0. I've plotted the surface charge below (note that it is negative).